Narzędzia użytkownika
Narzędzia witryny
Pasek boczny
en:statpqpl:warstwpl:mhpl
Spis treści
The Mantel-Haenszel method for several tables
The Mantel-Haenszel method for 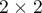 tables proposed by Mantel and Haenszel (1959)1) then it was extended by Mantel (1963)2). A wider review the development of these methods was carried out i.a. by Newman (2001)3).
tables proposed by Mantel and Haenszel (1959)1) then it was extended by Mantel (1963)2). A wider review the development of these methods was carried out i.a. by Newman (2001)3).
This method can be used in analysis 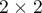 tables, that occur in several (
tables, that occur in several (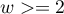 ) stratas constructed by confounding variable. For the next stratas (
) stratas constructed by confounding variable. For the next stratas (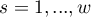 ) the
) the 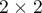 contingency tables for observed frequencies are created:
contingency tables for observed frequencies are created:
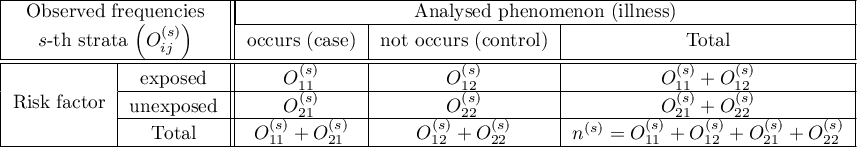
The settings window with the Mantel-Haenszel OR/RR can be opened in Statistics menu →Stratified analysis→Mantel-Haenszel OR/RR.
The Mantel-Haenszel Odds Ratio
If all tables (created by individual stratas) are homogeneous (the Chi-square test of homogeneity for the OR can check this condition), then, on the basis of these tables, the pooled odds ratio with the confidence interval can be designated. Such odds ratio, is a weighted mean for an odds ratio designated for the individual stratas. The usage of the weighted method, proposed by Mantel and Haenszel allows to include the contribution of the strata weights. Each strata has an influence on the pooled odds ratio (the greater size of the strata, the greater weight and the greater influence on the pooled odds ratio).
Weights for individual stratas are designated according to the following formula:
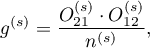 and the Mantel-Haenszel odds ratio:
and the Mantel-Haenszel odds ratio:
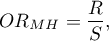
where:
 ,
,
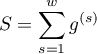 .
.
The confidence interval for 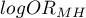 is designated on the basis of the standard error (RGB – Robins-Breslow-Greenland4)5) calculated according to the following formula:
is designated on the basis of the standard error (RGB – Robins-Breslow-Greenland4)5) calculated according to the following formula:
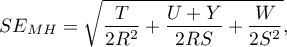
where:
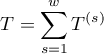 ,\qquad
,\qquad
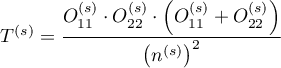 ,
,
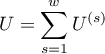 ,\qquad
,\qquad
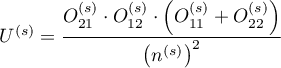 ,
,
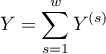 ,\qquad
,\qquad
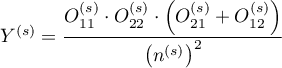 ,
,
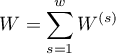 ,\qquad
,\qquad
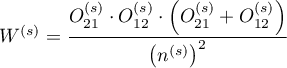 .
.
The Mantel-Haenszel Chi-square test for the 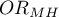
The Mantel-Haenszel Chi-square test for the 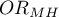 is used in the hypothesis verification about the significance of designated odds ratio (
is used in the hypothesis verification about the significance of designated odds ratio (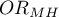 ). It should be calculated for large frequencies, i.e. when both conditions of the so-called „rule 5” are satisfied:
). It should be calculated for large frequencies, i.e. when both conditions of the so-called „rule 5” are satisfied:
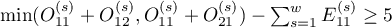 for all the stratas
for all the stratas 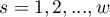 ,
,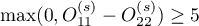 for all the stratas
for all the stratas 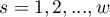 .
.
When there are zero values in the table, a continuity adjustment (increasing the counts by a value of 0.5) is applied to both the observed counts and the expected counts.
Hypotheses:
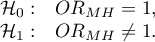
The test statistic is defined by:
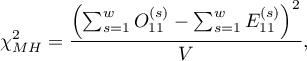
where:
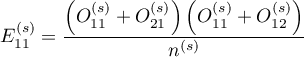 are the expected frequencies in the first contingency table cell, for the individual stratas
are the expected frequencies in the first contingency table cell, for the individual stratas 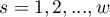 ,
,
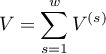 ,
,
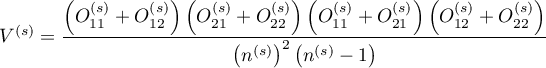 .
.
This statistic asymptotically (for large frequencies) has the Chi-square distribution with 1 degree of freedom.
The p-value, designated on the basis of the test statistic, is compared with the significance level  :
:

The Chi-square test of homogeneity for the 
The Chi-square test of homogeneity for the  is used in the hypothesis verification that the variable, creating stratas, is the modifying effect, i.e. it influences on the designated odds ratio in the manner that, the odds ratios are significant different for individual stratas.
is used in the hypothesis verification that the variable, creating stratas, is the modifying effect, i.e. it influences on the designated odds ratio in the manner that, the odds ratios are significant different for individual stratas.
Hypotheses:
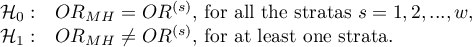
The test statistic (Breslow-Day (1980)6), Tarone (1985)arone (1985)7)8)) is defined by:

where:
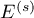 is solution to the quadratic equation:
is solution to the quadratic equation:
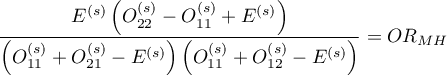 ,
,
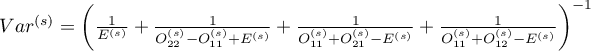 .
.
This statistic asymptotically (for large frequencies) has the Chi-square distribution with the number of degrees of freedom calculated using the formula:  .
.
The p-value, designated on the basis of the test statistic, is compared with the significance level  :
:

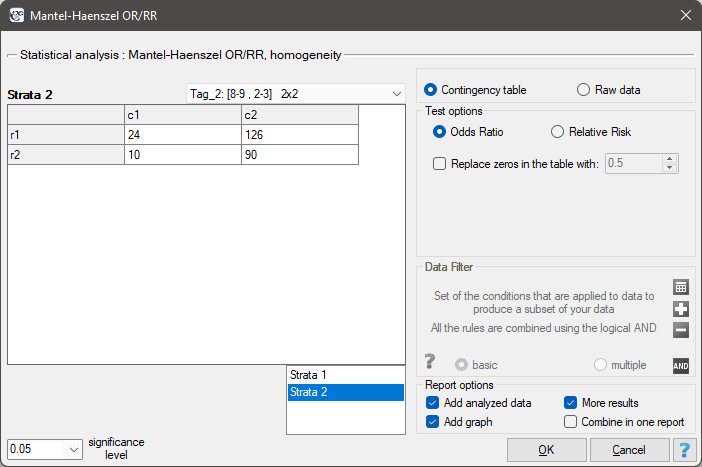
EXAMPLE (leptospirosis.pqs file)
The following table presents hypothetical poll results, conducted among inhabitants of a city and village (the village is treated as a risk factor) in West India. The poll aim was to detect risk factors of leptospirosis9). The occurrence of leptospirosis antibodies is a indirect evidence about infection.
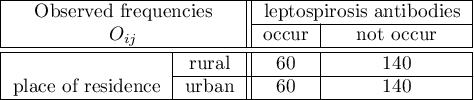
The odds of the occurrence of leptospirosis antibodies, among inhabitants of the city and the village, is the same (OR=1). Let's include gender in the analysis and check what odds will be then. The sample has to be divided into 2 stratas, because of gender (they are marked in a file as a saved selection):
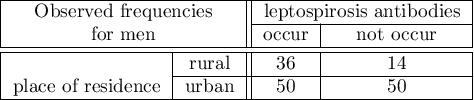

Gender is associated with both factors (the occurrence of leptospirosis anibodies and the residence in West India). This is a significant factor. Its ignorance can lead to errors in results.
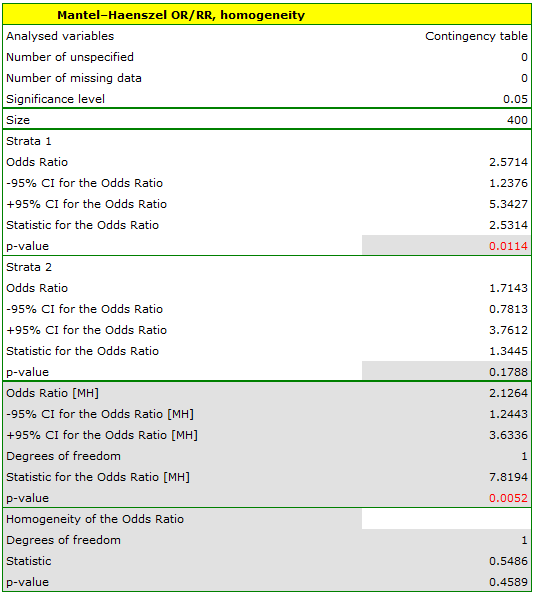
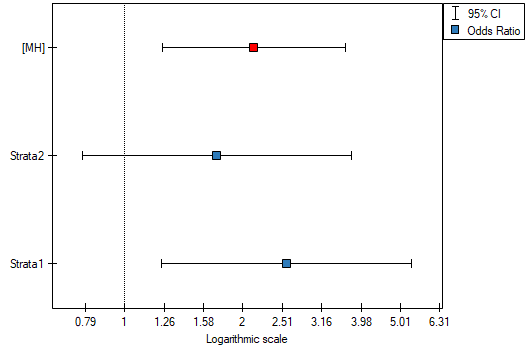
The odds of the occurrence of leptospirosis antibodies is larger among village inhabitants, both among women (OR[95%CI]=2.57[1.24, 5.34]) and men (OR[95%CI]=1.71[0.78, 3.76]). The tables are homogeneous (p=0.4589). Thus, we can use the calculated odds ratio, which is mutual for both tables (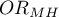 [95%CI]=2.13[1.24, 3.65]). Finally, the obtained result indicates that the odds of the occurrence of leptospirosis antibodies is significantly greater among village inhabitants (p=0.0052).
[95%CI]=2.13[1.24, 3.65]). Finally, the obtained result indicates that the odds of the occurrence of leptospirosis antibodies is significantly greater among village inhabitants (p=0.0052).
2022/02/09 12:56
The Mantel-Haenszel Relative Risk
If all tables (created by individual stratas) are homogeneous (the Chi-square test of homogeneity for the RR), can check this condition), then, on the basis of these tables, the pooled relative risk with the confidence interval can be designated. Such relative risk is a weighted mean for a relative risk designated for the individual stratas. The usage of the weighted method, proposed by Mantel and Haenszel allows to include the contribution of the strata weights. Each strata of the input has an influence on the pooled relative risk construction (the greater size of the strata, the greater weight and the greater influence on the pooled relative risk).
Weights for individual stratas are designated according to the following formula:

and the Mantel-Haenszel relative risk:
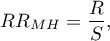
where:
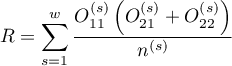 ,
,
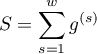 .
.
The confidence interval for 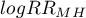 is designated on the basis of the standard error calculated according to the following formula:
is designated on the basis of the standard error calculated according to the following formula:

where:
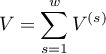 ,
,
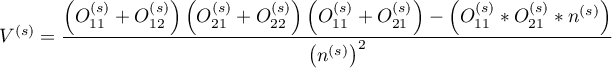 .
.
The Manel-Hanszel Chi-square test for the 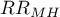
The Mantel-Haenszel Chi-square test for the 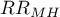 is used in the hypothesis verification about the significance of designated relative risk (
is used in the hypothesis verification about the significance of designated relative risk (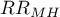 ). It should be calculated for large frequencies, in a contingency table.
). It should be calculated for large frequencies, in a contingency table.
Hypotheses:
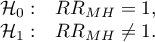
The test statistic is defined by:
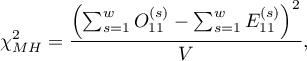
where:
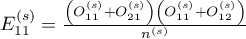 are the expected frequencies in the first contingency table cell, for individual stratas
are the expected frequencies in the first contingency table cell, for individual stratas 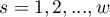 .
.
This statistic asymptotically (for large frequencies) has the Chi-square distribution with 1 degree of freedom.
The p-value, designated on the basis of the test statistic, is compared with the significance level  :
:

The Chi-square test of homogeneity for the 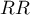
The Chi-square test of homogeneity for the 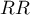 is used in the hypothesis verification that the variable creating stratas, is the modifying effect, i.e. it influences on the designated relative risk in the manner that, the relative risks are significant different for individual stratas.
is used in the hypothesis verification that the variable creating stratas, is the modifying effect, i.e. it influences on the designated relative risk in the manner that, the relative risks are significant different for individual stratas.
Hypotheses:
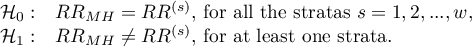
The test statistic, using weighted least squares method, is defined by:

where:
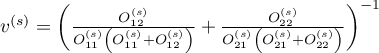 .
.
This statistic asymptotically (for large frequencies) has the Chi-square distribution with the number of degrees of freedom calculated using the formula: 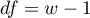 .
.
The p-value, designated on the basis of the test statistic, is compared with the significance level  :
:

2022/02/09 12:56
1)
, 2)
Mantel N. (1963), Chi-square tests with one degree of freedom: Extensions of the Mantel-Haenszel procedure. J. Am. Statist. Assoc., 58, 690-700
3)
Newman S.C.(2001), Biostatistical Methods in Epidemiology. 2nd ed. New York: John Wiley
4)
Robins, J., Breslow, N., and Greenland S. (1986), Estimators of the Mantel–Haenszel variance consistent in both sparse data and large-strata limiting models. Biometrics 42, 311–323
5)
Robins, J., Greenland S. and Breslow, N.E. (1986), A general estimator for the variance of the Mantel–Haenszel odds ratio. American Journal of Epidemiology 124, 719–723
6)
Breslow N.E., Day N.E. (1980), Statistical Methods in Cancer Research: Vol. I - The Analysis of Case-Control Studies. Lyon: International Agency for Research on Cancer
7)
Breslow N.E. (1996), Statistics in epidemiology: the case-control study', Journal of the American Statistical Association, 91, 14-28
8)
Tarone R.E. (1985), On heterogeneity tests based on efficient scores. Biometrika 72, 91–95
9)
Betty R. Kirkwood and Jonathan A. C. Sterne (2003), Medical Statistics (2nd ed.). Meassachusetts: Blackwell Science, 177-188, 240-248
en/statpqpl/warstwpl/mhpl.txt · ostatnio zmienione: 2022/02/13 18:06 przez admin
Narzędzia strony
Wszystkie treści w tym wiki, którym nie przyporządkowano licencji, podlegają licencji: CC Attribution-Noncommercial-Share Alike 4.0 International

